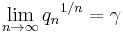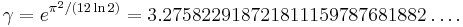Lévy's constant

In mathematics Lévy's constant (sometimes known as the Khinchin–Lévy constant) occurs in an expression for the asymptotic behaviour of the denominators of the convergents of continued fractions.[1] In 1935, the Soviet mathematician Aleksandr Khinchin showed[2] that the denominators qn of the convergents of the continued fraction expansions of almost all real numbers satisfy

\lim_{n \to \infty}{q_n}^{1/n}= \gamma

for some constant γ. Soon afterward, in 1936, the French mathematician Paul Lévy found[3] the explicit expression for the constant, namely

\gamma = e^{\pi^2/(12\ln2)} = 3.275822918721811159787681882\ldots.

The term "Lévy's constant" is sometimes used to refer to 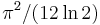\pi^2/(12\ln2) (the logarithm of the above expression), which is approximately equal to 1.1865691104….

The base-10 logarithm of Lévy's constant, which is approximately 0.51532941…, is half of the reciprocal of the limit in Lochs' theorem.

See also

References

  1. ^ A. Ya. Khinchin; Herbert Eagle (transl.) (1997), Continued fractions, Courier Dover Publications, p. 66, ISBN 9780486696300, http://books.google.com/books?id=R7Fp8vytgeAC&pg=PA66 
  2. ^ [Reference given in Dover book] "Zur metrischen Kettenbruchtheorie," Compositio Matlzematica, 3, No.2, 275–285 (1936).
  3. ^ [Reference given in Dover book] P. Levy, Théorie de l'addition des variables aléatoires, Paris, 1937, p. 320.

External links